07th May 2019 @ 12 min read
The Avogadro constant or (the Avogadro number earlier) is the number of elementary units in one mole of any substance. The Avogadro constant is denoted as NA. It has the dimension of the reciprocal amount of substance (mol−1). The approximate value of NA is 6.022 × 1023 mol−1. This means one mole of any substance contains 6.022 × 1023 elementary particles. The Avogadro constant is named after Italian scientist Amedeo Avogadro.
The full name of Avogadro was very long. He was born as Lorenzo Romano Amedeo Carlo Avogadro di Quaregna e di Cerreto. Due to his nobility, he served as the Count of Quaregna and Cerreto. Facts about Amedeo Avogadro 2: the molecular theory. In tribute to him, the number of elementary entities in 1 mole of a substance, 179000000♠6.02214179×10, is known as the Avogadro constant, one of the seven SI base units and represented by NA. Avogadro was born at Turin to a.
These elementary units in one mole can be anything like atoms, molecules, ions, electrons, protons, neutrons, particles of sand. So, when we say one mole of sodium chloride, it means 6.022 × 1023 molecules of sodium chloride.
Values of Avogadro's Constant
The value of the Avogadro constant is revised over a period of time. As of the 2019 redefinition of the SI base units, the value of the Avogadro constant is fixed to 6.02214076×1023mol−1. This is the exact value of the constant. The table below mentions the value of the constant in different units.
| Value | Unit |
|---|---|
| 6.022 140 76 × 1023 | mol−1 |
| 6.022 140 76 × 1026 | kmol−1 |
| 2.731 597 099 802 001 2 × 1026 | lb-mol−1 |
| 1.707 248 187 376 250 75 × 1025 | oz-mol−1 |
| 0.602 214 076 | mL mol−1 Å |
History of Avogadro's Constant
The Avogadro constant has a long history. The constant is named in honour of Avogadro, but he did not discover it. In 1811, Avogadro discovered the relationship between the volume of gas and the amount of gas through his experiments. He was the first to proposed the volume of a gas is directly proportional to the amount of the gas at constant pressure and temperature. This is today we call Avogadro's law or Avogadro's hypothesis. His work does not mention Avogadro's constant.
Perrin's Work
French Nobel Laureate Jean Baptiste Perrin estimated the Avogadro number with several methods. And he credited the naming of the number to Avogadro in 1909. Perrin named the number Avogadro's number, not Avogadro's constant. This name had continued till 1971. In 1971, the International System of Unit (SI) introduced a new quantity called Avogadro's constant. The Avogadro constant has the same numerical value as the Avogadro number, but they differ in the unit which will be explained later in this article.
Perrin defined the Avogadro number as the number of atoms in one gram of hydrogen (one gram-molecule). This definition was later revised to the number of atoms in 12 grams of carbon-12 (12C).
Loschmidt's Estimation
Before Perrin, Loschmidt also made a significant contribution to the number. Josef Loschmidt was an Austrian scientist who is notable for his work on estimation of the diameter of the molecules in the air. Through his method, it is possible to calculate the number density (the number of molecules or atoms per unit volume). This quantity is closely relative to the Avogadro constant. The relationship between them is discussed later in this article. The number density of an ideal gas is called as the Loschmidt constant. In many of German literature, these two constants are interchangeable. They can easily be distinguished from their units. The Avogadro constant is also denotated as L in honour of Loschmidt.
Other Efforts
- Of the most common names in scientific literature. Brief Life Sketch Amedeo Avogadro was born on 9th August, 1776, in an aristocratic family in Turin, Italy. His father was Count Filippo Avogadro and mother Anna Maria Vercelli. Befi tting his aristocracy, his full name was Lorenzo Romano Amedeo Carlo Avogadro, Conte di Queregna e di Cerreto.
- Amedeo Avogadro, in full Lorenzo Romano Amedeo Carlo Avogadro, conte di Quaregna e Cerreto, (born August 9, 1776, Turin, in the Kingdom of Sardinia and Piedmont Italy—died July 9, 1856, Turin), Italian mathematical physicist who showed in what became known as Avogadro's law that, under controlled conditions of temperature and pressure, equal volumes of gases contain an equal number of molecules.
Robert Millikan was an American physicist and Nobel Laureate. He successfully measured the charge on an electron in 1910. The electric charge per mole (the Faraday constant) of electrons had already known at that time. With the help of these two quantities, the electric charge on an electron and the Faraday constant, it is possible to calculate the number of electrons per mole. The value of this number of electrons per mole is the same as Avogadro's constant.
One of a modern method to estimate the value of the constant is X-ray crystallography. This method estimates the constant by determining the number of silicon atoms in a crystal cell, the volume per unit cell, and the molar volume.
The measurement of the accurate value of the Avogadro's constant is always troublesome. Over the period, the new methods were developed, and the Avogadro constant has continuously been improvised. From 2019, the international committee fixed the value of the Avogadro's constant exactly to 6.022 140 76 × 1023 mol−1.
2019 Redefinition and Prior Definition of Avogadro's Constant
As discussed above, the 2019 redefinition of the Avogadro constant is 6.022 140 76 × 1023 mol−1. The consequence of this redefinition is the prior definition of the constant is no longer valid. Before the 2019 redefinition, the value of the constant was defined as the amount of atoms presents in 12g of carbon-12 (12C). Also, because of the definition the molar mass constant (Mu) is no longer exactly equal to 1 g mol−1. Instead, it is approximately equal to 1 g mol−1. This is summarised in the table below.
| 2019 Redefinition | Prior to 2019 Redefinition |
|---|---|
| NA = 6.022 140 76 × 1023 mol−1 | The value of NA is the number of 12C atoms in 12 g of carbon-12. |
| The molar mass constant is approximately equal to 1 g mol−1 (Mu ≈ 1 g mol−1). | Mu is exactly equal to 1 g mol−1 (Mu = 1 g mol−1). |
Note: The difference in the value of the Avogadro constant before and after the 2019 definition is very small. The redefinition would not affect most of the calculations unless the high degree of precision is needed. For practical calculations, we can take NA = 6.022 × 1023 mol−1.
Avogadro's Constant and Mole
The Avogadro constant and the mole are related quantities. In fact, the Avogadro constant is defined in terms of the mole. The value of Avogadro's constant is the number of elementary units in one mole of any substance. The definition is universally true. The below equation establishes the relation between both.
Avogadro's Constant and Molar Mass
We can use the Avogadro constant to determine the mass of any atom if we know the molar mass of that atom. This statement is also true for molecules. The molar mass is the mass of one mole of a given sample. It is expressed in g mol−1. The relation between both is as follows:
where mi is the mass of atom i and Mi is molar mass of atom i.
Avogadro's Constant and Avogadro's Number
The Avogadro constant and the Avogadro number have the same numerical value. They only differ in the unit. The Avogadro number is a dimensionless quantity, but the Avogadro constant has the dimension of the reciprocal amount of substance (mol−1). The below table describes the same.
| Avogadro's Constant | Avogadro's Number |
|---|---|
| The constant has the unit of mol−1. | It is a dimensionless quantity. |
| It is denoted as NA. | We use N to denote the Avogadro number. |
| NA = 6.022 × 1023 mol−1 | NA = 6.022 × 1023 |
Avogadro's Constant and Boltzmann's constant
The Boltzmann constant is an important physical constant which plays a vital role in classical statistical mechanics. It is denoted as kB or simply k. The Avogadro constant is related to the Boltzmann constant by the gas constant R.
Avogadro's Constant and Loschmidt's Constant
The Loschmidt constant is the number density (the number of molecules per unit volume). For an ideal gas, the relationship between the Loschmidt constant and the Avogadro constant at STP (P0 = 1 atm, T0 = 273.15 K) is described in the equation below.
Avogadro's Constant and Faraday's Constant
The Faraday constant (F) is the Avogadro constant times the elementary charge (e).
Avogadro's Constant and Unified Mass Unit
The unified mass unit or the dalton (u) is the ratio of the molar mass constant (Mu) and the Avogadro constant.
where mu is the atomic mass constant.
The value precise value of Mu is 0.999 999 999 65(30) g mol−1. But for practical purposes, we can say Mu ≈ 1 g mol−1.
Examples
Example 1: To Determine Calcium Atoms
Statement: For 100 g of calcium in a beaker, calculate the number of calcium atoms in the beaker?
Solution: The molecular weight of calcium is 40.1 g mol−1. The number of moles of calcium in the beaker is
The number of calcium atoms in the beaker is calculated as:
Therefore, the number of calcium atoms is 1.50 × 1024.
Example 2: To Determine Total Molecules in Sodium Chlorine Solution
Statement: Consider 50.0 g of NaCl is dissolved in 200 g of water. Estimate the total molecules in the solution?
Solution: The molecular weight of NaCl and water is 58.44 g mol−1 and 18.01 g mol−1.
The moles of NaCl in 50.0 g:
The moles of H2O in 100 g:
When 1 mol of NaCl dissociates, 1 mol of Na+ and 1 mol of Cl− are formed. So, when 0.855 5 mol of NaCl dissociates, 0.855 5 mol of Na+ and 0.855 5 mol of Cl− are formed.
$underset{1,text{mol}}{ce{Na+}}$ + $underset{1,text{mol}}{ce{Cl-}}$}' alt='>Thus, the total number of moles after the dissociation is the sum of the moles of Na+, Cl−, and H2O.
The total number of molecules in the solution is
Therefore, the total number of moles in NaCl solution is 4.374 × 1024 mol.
Example 3: To Determine Mass of Sodium Atom
Statement: The molar mass of sodium-23 is 22.989 g mol−1. Calculates the mass of a sodium atom?
Solution: Let mNa and MNa be the atomic mass and molar mass of sodium-23. Thus, MNa = 22.989 g mol−1.
Now, mNa can be determined using the formula below.
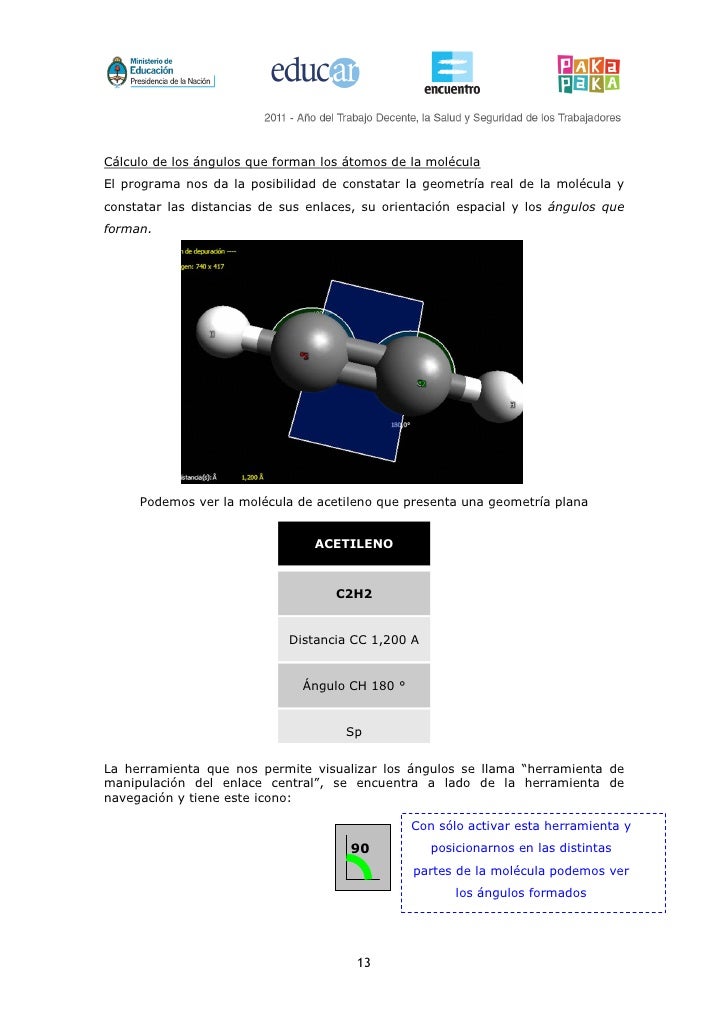
Therefore, the mass of a sodium-23 atom is 3.817 × 10−23 g.
Example 4: To Determine Molecular Mass of Iodine gas
Full Name Of Avogadro
Statement: The atomic mass of iodine is 126.9 g mol−1. Determine the molecular mass of iodine gas?
Solution: The iodine gas is a diatomic gas. The molecular formula is I2. So, the molar mass of I2 is twice the molar mass of I.
Now, mI2 can be determined as:
Therefore, the mass of a iodine molecule is 4.208 × 10−22 g.
Associated Articles
If you appreciate our work, consider supporting us on ❤️ patreon.- 4
- cite
- response
Copy Article Cite
'Avogadro's Constant' ChemistryGod, 07th May 2019, https://chemistrygod.com/avogadro-constant/29th Oct 2019

Learning Objectives
- To understand the relationships among pressure, temperature, volume, and the amount of a gas.
Early scientists explored the relationships among the pressure of a gas (P) and its temperature (T), volume (V), and amount (n) by holding two of the four variables constant (amount and temperature, for example), varying a third (such as pressure), and measuring the effect of the change on the fourth (in this case, volume). The history of their discoveries provides several excellent examples of the scientific method.
The Relationship between Pressure and Volume: Boyle's Law
As the pressure on a gas increases, the volume of the gas decreases because the gas particles are forced closer together. Conversely, as the pressure on a gas decreases, the gas volume increases because the gas particles can now move farther apart. Weather balloons get larger as they rise through the atmosphere to regions of lower pressure because the volume of the gas has increased; that is, the atmospheric gas exerts less pressure on the surface of the balloon, so the interior gas expands until the internal and external pressures are equal.
The Irish chemist Robert Boyle (1627–1691) carried out some of the earliest experiments that determined the quantitative relationship between the pressure and the volume of a gas. Boyle used a J-shaped tube partially filled with mercury, as shown in Figure (PageIndex{1}). In these experiments, a small amount of a gas or air is trapped above the mercury column, and its volume is measured at atmospheric pressure and constant temperature. More mercury is then poured into the open arm to increase the pressure on the gas sample. The pressure on the gas is atmospheric pressure plus the difference in the heights of the mercury columns, and the resulting volume is measured. This process is repeated until either there is no more room in the open arm or the volume of the gas is too small to be measured accurately. Data such as those from one of Boyle's own experiments may be plotted in several ways (Figure (PageIndex{2})). A simple plot of (V) versus (P) gives a curve called a hyperbola and reveals an inverse relationship between pressure and volume: as the pressure is doubled, the volume decreases by a factor of two. This relationship between the two quantities is described as follows:
[PV = rm constant label{6.2.1}]
Dividing both sides by (P) gives an equation illustrating the inverse relationship between (P) and (V):
[V=dfrac{rm const.}{P} = {rm const.}left(dfrac{1}{P}right) label{6.2.2}]
or
[V propto dfrac{1}{P} label{6.2.3}]
where the ∝ symbol is read 'is proportional to.' A plot of V versus 1/P is thus a straight line whose slope is equal to the constant in Equation 6.2.1 and Equation 6.2.3. Dividing both sides of Equation 6.2.1 by V instead of P gives a similar relationship between P and 1/V. The numerical value of the constant depends on the amount of gas used in the experiment and on the temperature at which the experiments are carried out. This relationship between pressure and volume is known as Boyle's law, after its discoverer, and can be stated as follows: At constant temperature, the volume of a fixed amount of a gas is inversely proportional to its pressure.
Full Name Of Avogadro
The Relationship between Temperature and Volume: Charles's Law
Hot air rises, which is why hot-air balloons ascend through the atmosphere and why warm air collects near the ceiling and cooler air collects at ground level. Because of this behavior, heating registers are placed on or near the floor, and vents for air-conditioning are placed on or near the ceiling. The fundamental reason for this behavior is that gases expand when they are heated. Because the same amount of substance now occupies a greater volume, hot air is less dense than cold air. The substance with the lower density—in this case hot air—rises through the substance with the higher density, the cooler air.
The first experiments to quantify the relationship between the temperature and the volume of a gas were carried out in 1783 by an avid balloonist, the French chemist Jacques Alexandre César Charles (1746–1823). Charles's initial experiments showed that a plot of the volume of a given sample of gas versus temperature (in degrees Celsius) at constant pressure is a straight line. Similar but more precise studies were carried out by another balloon enthusiast, the Frenchman Joseph-Louis Gay-Lussac (1778–1850), who showed that a plot of V versus T was a straight line that could be extrapolated to a point at zero volume, a theoretical condition now known to correspond to −273.15°C (Figure (PageIndex{3})).A sample of gas cannot really have a volume of zero because any sample of matter must have some volume. Furthermore, at 1 atm pressure all gases liquefy at temperatures well above −273.15°C. Note from part (a) in Figure (PageIndex{3}) that the slope of the plot of V versus T varies for the same gas at different pressures but that the intercept remains constant at −273.15°C. Similarly, as shown in part (b) in Figure (PageIndex{3}), plots of V versus T for different amounts of varied gases are straight lines with different slopes but the same intercept on the T axis.
The significance of the invariant T intercept in plots of V versus T was recognized in 1848 by the British physicist William Thomson (1824–1907), later named Lord Kelvin. He postulated that −273.15°C was the lowest possible temperature that could theoretically be achieved, for which he coined the term absolute zero (0 K).
We can state Charles's and Gay-Lussac's findings in simple terms: At constant pressure, the volume of a fixed amount of gas is directly proportional to its absolute temperature (in kelvins). This relationship, illustrated in part (b) in Figure (PageIndex{3}) is often referred to as Charles's law and is stated mathematically as
[V ={rm const.}; T label{6.2.4}]
or
[V propto T label{6.2.5}]
with temperature expressed in kelvins, not in degrees Celsius. Charles's law is valid for virtually all gases at temperatures well above their boiling points.
The Relationship between Amount and Volume: Avogadro's Law
We can demonstrate the relationship between the volume and the amount of a gas by filling a balloon; as we add more gas, the balloon gets larger. The specific quantitative relationship was discovered by the Italian chemist Amedeo Avogadro, who recognized the importance of Gay-Lussac's work on combining volumes of gases. In 1811, Avogadro postulated that, at the same temperature and pressure, equal volumes of gases contain the same number of gaseous particles (Figure (PageIndex{4})). This is the historic 'Avogadro's hypothesis.'
A logical corollary to Avogadro's hypothesis (sometimes called Avogadro's law) describes the relationship between the volume and the amount of a gas: At constant temperature and pressure, the volume of a sample of gas is directly proportional to the number of moles of gas in the sample. Stated mathematically,
[V ={rm const.} ; (n) label{6.2.6}]
or

Therefore, the mass of a sodium-23 atom is 3.817 × 10−23 g.
Example 4: To Determine Molecular Mass of Iodine gas
Full Name Of Avogadro
Statement: The atomic mass of iodine is 126.9 g mol−1. Determine the molecular mass of iodine gas?
Solution: The iodine gas is a diatomic gas. The molecular formula is I2. So, the molar mass of I2 is twice the molar mass of I.
Now, mI2 can be determined as:
Therefore, the mass of a iodine molecule is 4.208 × 10−22 g.
Associated Articles
If you appreciate our work, consider supporting us on ❤️ patreon.- 4
- cite
- response
Copy Article Cite
'Avogadro's Constant' ChemistryGod, 07th May 2019, https://chemistrygod.com/avogadro-constant/29th Oct 2019
Learning Objectives
- To understand the relationships among pressure, temperature, volume, and the amount of a gas.
Early scientists explored the relationships among the pressure of a gas (P) and its temperature (T), volume (V), and amount (n) by holding two of the four variables constant (amount and temperature, for example), varying a third (such as pressure), and measuring the effect of the change on the fourth (in this case, volume). The history of their discoveries provides several excellent examples of the scientific method.
The Relationship between Pressure and Volume: Boyle's Law
As the pressure on a gas increases, the volume of the gas decreases because the gas particles are forced closer together. Conversely, as the pressure on a gas decreases, the gas volume increases because the gas particles can now move farther apart. Weather balloons get larger as they rise through the atmosphere to regions of lower pressure because the volume of the gas has increased; that is, the atmospheric gas exerts less pressure on the surface of the balloon, so the interior gas expands until the internal and external pressures are equal.
The Irish chemist Robert Boyle (1627–1691) carried out some of the earliest experiments that determined the quantitative relationship between the pressure and the volume of a gas. Boyle used a J-shaped tube partially filled with mercury, as shown in Figure (PageIndex{1}). In these experiments, a small amount of a gas or air is trapped above the mercury column, and its volume is measured at atmospheric pressure and constant temperature. More mercury is then poured into the open arm to increase the pressure on the gas sample. The pressure on the gas is atmospheric pressure plus the difference in the heights of the mercury columns, and the resulting volume is measured. This process is repeated until either there is no more room in the open arm or the volume of the gas is too small to be measured accurately. Data such as those from one of Boyle's own experiments may be plotted in several ways (Figure (PageIndex{2})). A simple plot of (V) versus (P) gives a curve called a hyperbola and reveals an inverse relationship between pressure and volume: as the pressure is doubled, the volume decreases by a factor of two. This relationship between the two quantities is described as follows:
[PV = rm constant label{6.2.1}]
Dividing both sides by (P) gives an equation illustrating the inverse relationship between (P) and (V):
[V=dfrac{rm const.}{P} = {rm const.}left(dfrac{1}{P}right) label{6.2.2}]
or
[V propto dfrac{1}{P} label{6.2.3}]
where the ∝ symbol is read 'is proportional to.' A plot of V versus 1/P is thus a straight line whose slope is equal to the constant in Equation 6.2.1 and Equation 6.2.3. Dividing both sides of Equation 6.2.1 by V instead of P gives a similar relationship between P and 1/V. The numerical value of the constant depends on the amount of gas used in the experiment and on the temperature at which the experiments are carried out. This relationship between pressure and volume is known as Boyle's law, after its discoverer, and can be stated as follows: At constant temperature, the volume of a fixed amount of a gas is inversely proportional to its pressure.
Full Name Of Avogadro
The Relationship between Temperature and Volume: Charles's Law
Hot air rises, which is why hot-air balloons ascend through the atmosphere and why warm air collects near the ceiling and cooler air collects at ground level. Because of this behavior, heating registers are placed on or near the floor, and vents for air-conditioning are placed on or near the ceiling. The fundamental reason for this behavior is that gases expand when they are heated. Because the same amount of substance now occupies a greater volume, hot air is less dense than cold air. The substance with the lower density—in this case hot air—rises through the substance with the higher density, the cooler air.
The first experiments to quantify the relationship between the temperature and the volume of a gas were carried out in 1783 by an avid balloonist, the French chemist Jacques Alexandre César Charles (1746–1823). Charles's initial experiments showed that a plot of the volume of a given sample of gas versus temperature (in degrees Celsius) at constant pressure is a straight line. Similar but more precise studies were carried out by another balloon enthusiast, the Frenchman Joseph-Louis Gay-Lussac (1778–1850), who showed that a plot of V versus T was a straight line that could be extrapolated to a point at zero volume, a theoretical condition now known to correspond to −273.15°C (Figure (PageIndex{3})).A sample of gas cannot really have a volume of zero because any sample of matter must have some volume. Furthermore, at 1 atm pressure all gases liquefy at temperatures well above −273.15°C. Note from part (a) in Figure (PageIndex{3}) that the slope of the plot of V versus T varies for the same gas at different pressures but that the intercept remains constant at −273.15°C. Similarly, as shown in part (b) in Figure (PageIndex{3}), plots of V versus T for different amounts of varied gases are straight lines with different slopes but the same intercept on the T axis.
The significance of the invariant T intercept in plots of V versus T was recognized in 1848 by the British physicist William Thomson (1824–1907), later named Lord Kelvin. He postulated that −273.15°C was the lowest possible temperature that could theoretically be achieved, for which he coined the term absolute zero (0 K).
We can state Charles's and Gay-Lussac's findings in simple terms: At constant pressure, the volume of a fixed amount of gas is directly proportional to its absolute temperature (in kelvins). This relationship, illustrated in part (b) in Figure (PageIndex{3}) is often referred to as Charles's law and is stated mathematically as
[V ={rm const.}; T label{6.2.4}]
or
[V propto T label{6.2.5}]
with temperature expressed in kelvins, not in degrees Celsius. Charles's law is valid for virtually all gases at temperatures well above their boiling points.
The Relationship between Amount and Volume: Avogadro's Law
We can demonstrate the relationship between the volume and the amount of a gas by filling a balloon; as we add more gas, the balloon gets larger. The specific quantitative relationship was discovered by the Italian chemist Amedeo Avogadro, who recognized the importance of Gay-Lussac's work on combining volumes of gases. In 1811, Avogadro postulated that, at the same temperature and pressure, equal volumes of gases contain the same number of gaseous particles (Figure (PageIndex{4})). This is the historic 'Avogadro's hypothesis.'
A logical corollary to Avogadro's hypothesis (sometimes called Avogadro's law) describes the relationship between the volume and the amount of a gas: At constant temperature and pressure, the volume of a sample of gas is directly proportional to the number of moles of gas in the sample. Stated mathematically,
[V ={rm const.} ; (n) label{6.2.6}]
or
[V propto.n text{@ constant T and P} label{6.2.7}]
About Avogadro
This relationship is valid for most gases at relatively low pressures, but deviations from strict linearity are observed at elevated pressures.
Note
For a sample of gas,
- V increases as P decreases (and vice versa)
- V increases as T increases (and vice versa)
- V increases as n increases (and vice versa)
The relationships among the volume of a gas and its pressure, temperature, and amount are summarized in Figure (PageIndex{5}). Volume increases with increasing temperature or amount but decreases with increasing pressure.
Avogadro Name
Summary
The volume of a gas is inversely proportional to its pressure and directly proportional to its temperature and the amount of gas. Boyle showed that the volume of a sample of a gas is inversely proportional to its pressure (Boyle's law), Charles and Gay-Lussac demonstrated that the volume of a gas is directly proportional to its temperature (in kelvins) at constant pressure (Charles's law), and Avogadro postulated that the volume of a gas is directly proportional to the number of moles of gas present (Avogadro's law). Plots of the volume of gases versus temperature extrapolate to zero volume at −273.15°C, which is absolute zero (0 K), the lowest temperature possible. Charles's law implies that the volume of a gas is directly proportional to its absolute temperature.

